Using Algebra and Geometry

Question      

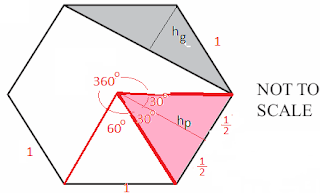
In the regular hexagon below, compare the shaded area to the entire area. 

 There appear to be three tasks.

·         Find the grey area. 

·         Find the total area.

·         Compare the two results.

Where to start? It is often a good idea to make a rough sketch. 

Central congruent triangles

We don’t know if the hexagon has sides of 3 cm or 2 miles or ….  Actually it doesn’t matter.  Each of the sides is 1s.  Partition the hexagon into 6 identical equilateral triangles (One has been shaded pink).  The apothem (or “radius”) is the height of the pink triangle, h_p.

The area of the pink triangle can be found using A= 1/2  (side)(side)  sin (included angle).  Your calculation should show the area of a pink triangle is √3/4. Therefore the area of the entire hexagon is  6√3/4 .

The grey area

Now consider the grey triangle.  The sum of the interior angles is  180(n - 2)°=720°. Therefore one interior angle is 120°. The area of the grey triangle is 1/2  (side)(side)  sin (included angle) =                   1/2 (1)(1)  sin (120°) = √3/4.  

                                
Solution

The ratio of  kX to X  is √3/4  :  6√3/4  so k is 1 ∶ 6 =  1/6 .

Aha!

Is the figure below a cube or a hexagon?   Actually we can consider the hexagon to be the ‘projection’  (or shadow) of a cube.  Immediately, we see the shaded area is one-sixth of the total area.

Input: A cube(3-D shape) casts a shadow on a wall. Output: A regular hexagon (2-D shape) is partitioned into 6 congruent triangles.  Thanks to students S.H. and A.L. for partitioning the hexagon into congruent triangles.

Conclusion

When you have the answer, don't stop. A hard-won solution may prompt additional insight.